Stability of Permanent Magnets

Claims that magnets deliver energy by losing their strength

In the past, several magnet motor inventors have claimed that the permanent magnets in their machines deliver energy into those machines by losing their strength over time, i.e. that the magnets themselves are not stable, and so are a source of energy from that loss of strength. David Porter makes this claim at 1:04:20 in the video cited previously, at https://www.youtube.com/watch?v=PfEbCBcddQw 

Yasunori Takahashi made a similar claim, to Chris Tinsley, about his Self-Generating Motor:—

"I asked Mr. Takahashi about this scooter. Was it over-unity? Seemingly not. Apparently the magnets lose strength at about 3% per year, and Takahashi claims this to be the source of the energy. But he had a glint in his eye when he said it. When I said, "No, it isn't," he grinned."

Ref: "Chris Tinsley: Travels in the New Energy Age," Infinite Energy magazine Vol 1, No 5/6, 1996, p29.

Such claims imply that permanent magnets are "used up" as if they were, say, "a gallon of petrol or a torch battery" (we'll meet that expression again, below).

Ridiculous — in theory and practise

This is a ridiculous concept, both theoretically and practically, as will now be shown. Why some inventors have made such claims, which they must know will reduce their credibility, is yet another of the many unanswered questions that swirl around the subject of over-unity magnet motors.

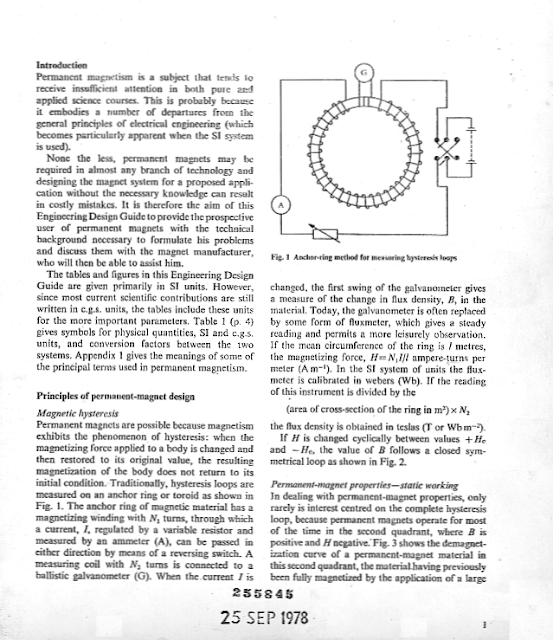
Engineering Design Guide

There is some useful information on-line about magnet stability, e.g. at http://www.magnetsales.com/design/designg_frames/designg_2.htm However, I couldn't find what I was really looking for — the Engineering Design Guide for permanent magnets which I first found in my local Public Library, of all places. It was eliminated from there many years ago (but not before I had copied most of it). I don't have any way of identifying it now apart from its first page, reproduced above.

Quoting from page 11 of this Engineering Design Guide:—

"Stability of permanent magnets

The designer planning to use permanent magnets must be confident that each magnet will remain stable in its working environment, or that any changes will be predictable. When a fully magnetized magnet is removed from the magnetizer, the first immediate loss of magnetization continues on a logarithmic scale, subsequent periods of 10, 10², 10³, etc, times the interval over which the initial loss was measured resulting in equal changes. If the first rapid change is accelerated by stabilization after magnetization, then — assuming a fixed steady temperature — the magnet is likely to remain stable to within 0.01 per cent for many years.

The chief causes of magnetic instabilty are temperature changes, exposure to stray magnetic fields, and mechanical shock or vibration, although in each case the same underlying mechanism is responsible. When a magnet is fully magnetized, it is thermodynamically unstable with the least stable domains trying to return to a state of lower energy by domain boundary movement or reversals. If the magnet is subjected to stray magnetic fields, or if thermal agitation is increased by a rise in temperature, then these unstable domains are the first to revert to their equilibrium state, leading to a loss of magnetization in the magnet. If, after fully magnetizing, some flux reduction is deliberately brought about by the application of a small alternating field, the unstable domains are the ones which are affected and, since these have already relaxed, a subsequent increase in temperature will have a reduced effect. It follows that stabilization by alternating flux reduction is a good general insurance against further loss, but for the highest stability requirements, this should be followed by cycling through a temperature range slightly wider than that to which the magnet will be exposed in use. Similarly the magnet may be subjected to any other abnormal conditions it is likely to encounter (for example, excessive vibration) in order to minimize further losses from these causes before it is put into operation."

So, as long as a magnet is first subjected to any conditions tending to demagnetize it which are slightly in excess of those which it will encounter in use, it will remain stable indefinitely.


(BH)_max  or Energy Product calculation

Quoting again from the above Engineering Design Guide:—

"There is one particular working point on the demagnetization curve [of flux density B versus magnetizing force H] for which the product BH is a maximum. This maximum is referred to as (BH)_max and is a useful characteristic of the material. It has the dimensions of energy per unit volume (Jm^-3) and is sometimes called the energy product, although (BH)_max is actually numerically twice the available energy of the magnet. For this reason the use of this term is not recommended, particularly as a magnet is not used as a source of energy in the same way as, say, a gallon of petrol or a torch battery."

The amount of permanent magnetic material used in David Porter's or Yasunori Takahashi's motors has not been reported, but we can certainly say that the permanent magnets in the Kure Tekko motor must have weighed less than its total reported weight of 155 pounds, i.e. 70.307kg. If we (generously) use that figure, and (very generously) assume all magnets to have been samarium-cobalt with a density of 8250kg/m³, that would give a volume of 0.008522m³ for its total magnetic material.

At say 20MgOe = 159155 J/m³ for samarium-cobalt, that means the total available energy from the energy product of the Kure Tekko motor's magnets would be very generously estimated at ½ × 0.008522 × 159155 = 678.16 joules.

We also have a reported power output for the Kure Tekko motor of 45 hp = 33556.5 watts. So if energy was really being taken from the magnets themselves to run it, then at full power it would have exhausted itself in about two-hundredths of a second!